Magnetic Anomaly Maps Backup Material
IEEE/ION PLANS 2023, Monterey, CA
Aaron Nielsen
The views expressed in this article are those of the author and do not necessarily reflect the official policy or position of the United States Government, Department of Defense, United States Air Force or Air University.
Distribution A: Authorized for public release. Distribution is unlimited. Case No. 2023-0277.
Scalar magnetic potentials
Scalar Magnetic Potential \(\Phi_M\)
- If \(\vec{J} = 0\), then \(\nabla \times \vec{H} = 0\)
- \(\vec{H}(\vec{r}) = -\nabla \Phi_M(\vec{r})\)
- and since \(\nabla \cdot \vec{B} = 0 \rightarrow \nabla^2 \Phi_M(\vec{r}) = 0\)
- LaPlacian equation: \(\nabla^2 \Phi_M(\vec{r}) = 0\)
- Solutions are often referred to as harmonic (sins and cosins)
- This situation holds for many airborne problems
Scalar Potential Theory
A procedure to transform magnetic scalar potential \(\Phi_M\) in free space.
From Laplacian we can write (Green’s third identity) \[
\Phi_M(\vec{r}) = \frac{1}{4\pi} \int_S \left( \frac{1}{r} \frac{\partial \Phi_M}{\partial n} -
\Phi_M \frac{\partial}{\partial n} \frac{1}{r} \right) dS
\]
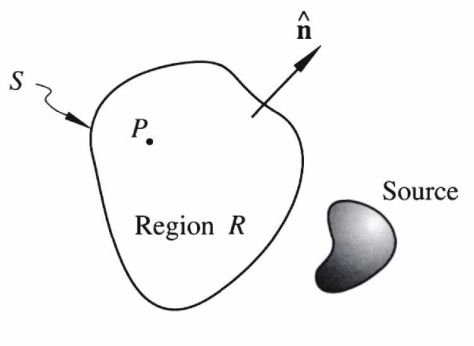
- No sources in region \(R\)
- Know \(\Phi_M\) on the surface \(S\) that bounds \(R\)
- We can find \(\Phi_M\) anywhere in \(R\)
- (Blakely 1995)
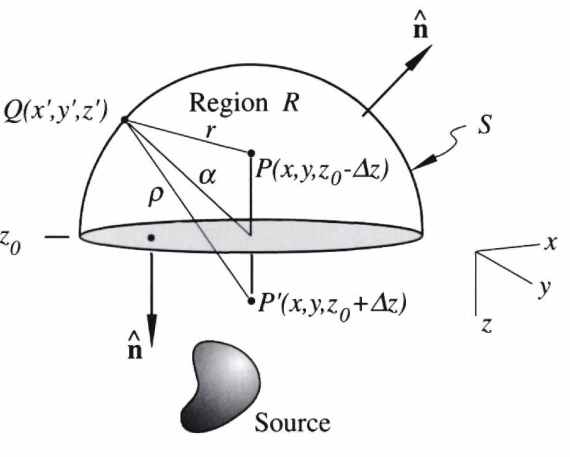
Hemispherical geometry - flat Earth model
- Think of Earth’s surface as an infinite flat plane, all sources below
- Measure the potential on a plane above the earth
- Other side of hemisphere is \(\infty\) away and therefore zero potential
- Potential anywhere inside can be computed
Fourier methods for potential theory
- Goal: find the potential \(\Phi_M\) on a plane parallel to a known plane
- Process: double integral over the surface of the known plane
- Ends up looking like a 2-D Fourier transform over spatial coordinates
- (Blakely 1995)


ION/IEEE PLANS MagNav Tutorial 2023
Blakely, Richard J. 1995. Potential Theory in Gravity and Magnetic Applications. Cambridge University Press.